February 17: Fractals and Branching in Embryonic Development
This lecture began with a bit more on dilation symmetry. The following text was in the lecture notes for Monday, February 13, but wasn't discussed that day:
Fractals are another interesting category of dilation symmetry. Each part of a fractal pattern is a miniature scale model of the entire pattern.
The branching pattern of the lungs are an example of a fractal. Embryologically, lungs form as two small out-pocketings of the wall of the endodermal tube. The epithelial walls of these out-pocketings fold actively (driven by contraction of cytoplasmic acto-myosin) so as to subdivide themselves into two pockets. Each of these pockets then repeats this process of subdivision into two, and this doubling process is repeated again and again, creating first 2, then 4, then 8 then 16, 32, 64 etc. out-pocketings. In humans, this process of repeated doubling continues until a million small epithelial pockets have been formed, each one of which then becomes a thin-walled alveolus. The lungs of all mammals have alveoli, but lung structures are significantly different in birds, reptiles and amphibians. For example, birds have "air capillaries", which are epithelial tubes that extend around in loops, so that air can flow continually in one direction, instead of in and out.
Other organs also form by sequential branching of epithelial tubes. Salivary glands, the pancreas, the liver, and ducts inside kidneys form this way. During this branching process, you could hardly distinguish between developing lungs, developing salivary glands, pancreas or kidneys. A time-lapse video of kidney development was shown in class, but cannot be incorporated into the course web pages, because I didn't make it, and a publisher owns the copyright.
video of a non-biological branching phenomenon (bubbles forming in a thin layer of gelatin, recorded in real time
The Mandelbrot set is one of many mathematical fractal patterns that are comparatively easy to generate by repeated recalculations. This bright-colored blobby pattern is a tiny part of the Mandelbrot set, which was generated on my Mac laptop by repeated squaring of complex numbers (those numbers that include the square root of minus one).
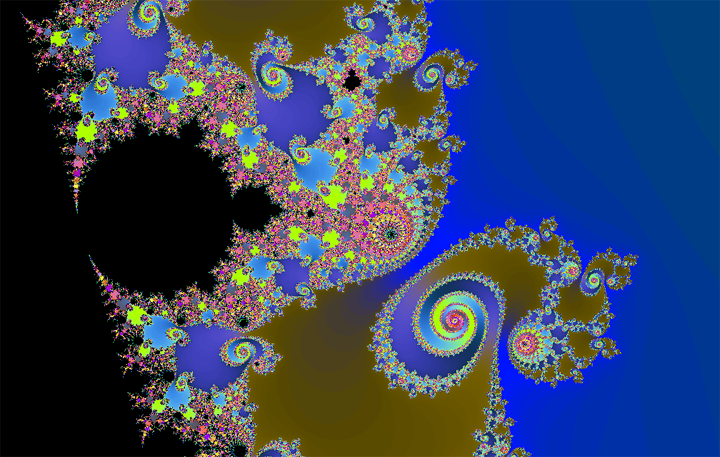
Repeated cubing will also generate fractal patterns, and so will repetition of other processes, whether mathematical or physical (as in the lungs). Tidal rivers and creeks develop fractal branching patterns. You can see pictures of them using Google Maps. Good examples are the rivers east of Elizabeth City and in Currituck County, North Carolina. The North River is a good example.

The lecture on February 17 continued with a discussion of somites and the notochord. This topic will be continued on February 20, and notes will be posted then.