Biology 441, Spring 2014: Lecture notes, January 17
How many kinds of symmetry can you find in the picture below?
Symmetry is the best unifying concept for shape and pattern generation:
1) Human oocytes have an infinite number of planes of reflection symmetry2) Developing embryos are reduced to just one plane of reflection symmetry
3) This remaining symmetry is then "broken" , by a cellular mechanism which depends on the 9-fold rotational symmetry of flagella.
4) Reduction of symmetry is called symmetry breaking, which is fundamental to development of animals (and plants), and sometimes is accomplished by gravity, locations of sperm entry or by amplification of random stimuli.
5) The segmentation of the spine, ribs and segmental nerves form displacement symmetry.
6) Driesch's "embryonic regulation" includes at least 8-fold dilation symmetry.
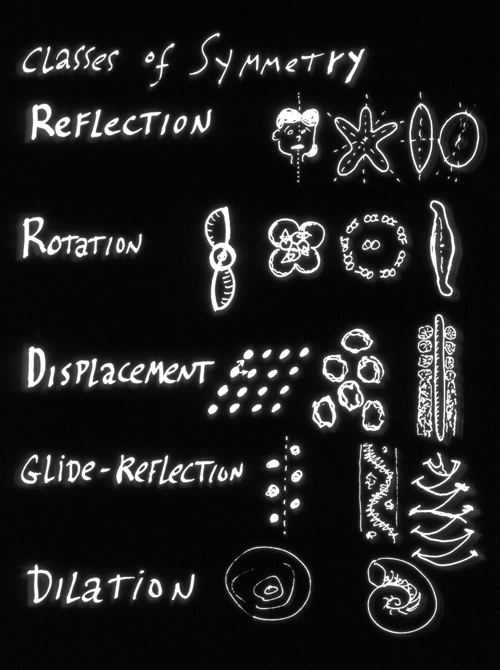
7) Each kind of symmetry is defined by some geometric operation (e.g. reflection, or rotation, or displacement, or dilation : which means magnification or shrinkage) which leaves an object looking the same as it did before.
8) Physical forces and other causes also have particular symmetries.
9) Effects have the same symmetries as their causes ("Curie's Principle.")
10)
Laws of conservation of energy, momentum, electric charge etc. turn out to be results of
symmetries of physical forces and other laws. For every symmetry, a conservation law...
This amazing fact is called "Emmy Noether's Theorem", but is Physics not Biology.

Lots of books have been written about how to apply symmetry to science.
Review: If twisting something leaves it looking the same as before...
Then we should say it has "twist symmetry."
For embryos to develop that symmetry, some force would have "twist symmetry".
If laws of nature had "twist symmetry", then there would be a law of conservation!
Please understand that there is no such thing as "twist symmetry"; it's just an example.
"There are more things in heaven and earth, Horatio, than are dreamt of in your Philosophy"
Shakespeare, Hamlet, Act One, Scene Five
For example: There are a lot more different kinds of symmetry, than just reflection symmetry. In fact there are more kinds of reflection symmetry than most people realize. Horatio didn't know!
Also, not just objects, but also forces have symmetries. Objects that are created by forces almost always have the same symmetry as the forces that cause them.
Soap bubbles have spherical symmetry because the air pressure inside pushes outward with the same force in every direction (Spherical Symmetry), ... and the tension in the soap film is also equal in strength in every direction.
Symmetries of crystals result from the symmetry of packing of molecules & ions.
The earth and the sun are spheres because the counterbalanced forces that shape them have the same strength in every direction. Notice that the forces are different in each of these cases: soap bubble, spherical balloon, earth, sun...
But forces that are equally strong in every direction (meaning they have spherical symmetry) cannot produce any shapes but spheres.
Please notice that oocytes of most species are spheres (Humans, birds, fish, sea urchins!
But not Flies (because their attachment to their "nurse cells" has only reflection symmetry).
Can you explain why human oocytes are spherical?
Even blastulas have almost spherical symmetry.
Axial Symmetry (which many people call "Radial Symmetry", but I prefer not to) is what you call it when an infinite number of planes of symmetry intersect along a line. For example, an ordinary drinking glass, and unbent pipe or rod, all have "Axial Symmetry."
In order for something to become cubic in shape (like salt crystals) some force needs to have cubic symmetry (which is a certain combination of reflection symmetries). In salt crystals, this special symmetry is contributed by the packing of the ions.
Crystallographers have organized their subject primarily around symmetry concepts, and relating the macroscopic shapes of crystals to the combinations of symmetrical packing obeyed by their component molecules or ions.
What sort of cause is needed to convert a spherical oocyte to an axially symmetrical blastula?
"Symmetry of a cause is a subgroup of the symmetry of the effect, or corresponds to it." This is called "Curie's Principle", named after Madame Curie's husband. Effects have the same combinations of symmetries as their causes (almost always) This applies to all sorts of things, including algebraic equations, and their graphed curves. Y = X squared, or to any even power, has a graph with a vertical plane of mirror image symmetry. That is because Y for any positive value of X has the same value as for the negative value of X. For equations like X=Y, in which x and y can be interchanged, then the graph has a diagonal plane of symmetry.
The equation X squared plus Y squared equals a constant gives a circle, because interchanging X with minus X, Y with minus Y, and X with Y all cause no change. From now on, you will be able to amaze your friends by knowing what symmetry the graph of an equation has to produce, won't you.
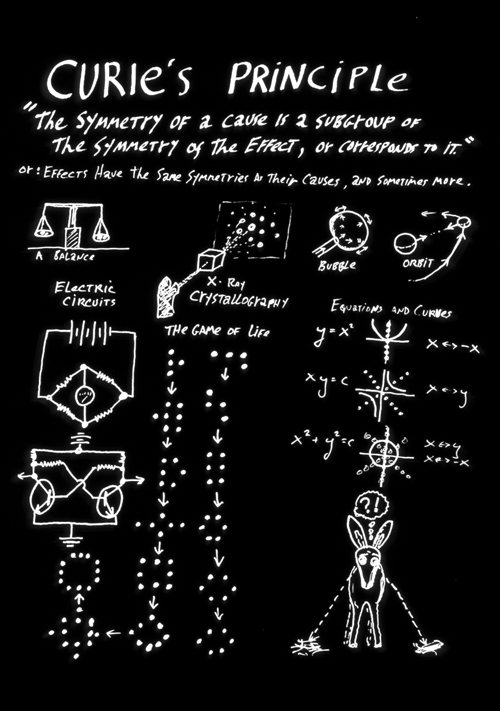
In X-ray Diffraction or in Electron Diffraction methods, the geometric patterns of spots produced is not a map of the submicroscopic molecules or ions in the crystal, but the diffraction pattern does have the same combination of symmetries.
The traditional style of weighing balance has mirror image symmetry for a reason.
In electronics, Wheatstone Bridges have two planes of symmetry for a reason.
Please don't panic if these things are not intuitively obvious.
Some medieval philosophers supposed that you could starve a donkey to death by putting it exactly half-way between two piles of hay. It is worth meditating on why this isn't true. Would it be true if hay repelled donkeys? Hint: maybe.
Also, the circuit diagram below the Wheatstone bridge is a classic transistor "flip-flop" that becomes stable when either of two electrical paths exist, and is the original memory device for computers.
Before we get back to embryos, I want to repeat some even more profound aspects of symmetry (which you won't be tested on in this course, but are pretty neat.)
______________________________________________________________________
Profound fact number one (That you won't be tested on)
"Emmy Noether's Theorem" which is named after a German woman mathematician who came as a refugee to the US and was a Professor at Bryn Mawr College, which is a Quaker women's college, about a dozen miles south-west of Philadelphia.The laws of conservation of momentum, angular momentum, electrical charge, energy & mass, and at least two others, are each based on a specific symmetry in laws of physics. If laws are unchanged by time displacement, then energy is conserved, etc. Symmetry with respect to quantum mechanical wave phase = conservation of charge.
Richard Feynman said, speaking very seriously, that Noether's Principle is the single most profound fact in all of physics.
 The Feynman Lectures on Physics are now available on line: http://feynmanlectures.caltech.edu/
______________________________________________________________________
The Feynman Lectures on Physics are now available on line: http://feynmanlectures.caltech.edu/
______________________________________________________________________
Profound fact number two (That you also won't be tested on)
"Geometric Duality"In every (true) theorem of either Euclidian Plane Geometry, Solid Geometry, or Projective Geometry, you can interchange certain pairs of words (point <-> line) and produce another theorem which is its "Dual", and which is also true. A French mathematician discovered this, and proved it mathematically, in the early 1800s, which resulted in hundreds of new geometric theorems being discovered (automatically!)
http://en.wikipedia.org/wiki/Duality_(projective_geometry)
http://mathworld.wolfram.com/DualityPrinciple.html ______________________________________________________________________
Remember, you won't be tested on this, either.
A third amazing aspect of symmetry:
If you take any electronic circuit, and replace every capacitance by an inductance, and also do the reverse, replace every inductance by a capacitance, then the new electric circuit will behave the same way as the original circuit (if the original was an amplifier, then the new circuit will also be an amplifier; and so on for every possible function.)
____________________________________________________________________
NOW BACK TO STUFF THAT YOU WILL BE TESTED ON
The Mathematician-Physicist names Ernst Weyl defined all forms of symmetry by saying that if there is something you can do to an object (like reflect it in a mirror), and the object looks the same afterward as it did before, then the object has whatever kind of symmetry as the action that left it seemingly unchanged (in this case, reflection symmetry).
So, for example, if it looks the same after you rotate it by 180 degrees then it has two fold rotation symmetry; if it looks the same if you rotate it by 120 degrees, then it has three-fold rotation symmetry.
If it looks the same after you magnify or shrink it, then it has dilation symmetry.
Please notice that dilation symmetry is connected to Driesch's embryonic regulation.
If Driesch had known more mathematics, then he might have decided that his discovery proved that embryonic shape-generating mechanisms have dilation symmetry. (In species with regulative development, at least; maybe also in mosaic species)
For example, in Dictyostelium and other cellular slime molds, the mechanisms that cause formation of stalks and spore masses have a huge amount of dilation symmetry. These shape-creating mechanisms also have axial symmetry (because the shapes of the stalk and the spore mass both have axial symmetry).


Pyramid of the Sun and Pyramid of the Moon, Teotihuacan, Mexico
These pyramids have reflectional and dilation symmetry.
In applied mathematics, dilation symmetry is called "Scaling" This is in the sense of scale models, like scale model electric trains, scale model ships and airplanes, etc.
The best-studied example of scaling in physics is explosions and "Mushroom Shaped Clouds". Clouds produced by explosions will produce the same shaped clouds over a range from the size of a small terrorist bomb (10 pounds, 50 pounds, etc.) up to many megaton hydrogen bombs. When I was a graduate student, a right-wing group set off a bomb a block from our apartment; and what the books say is true; the cloud was only a couple hundred feet wide and tall, but looked like news photographs of nuclear bombs. Actual mushrooms (Fungi, like you see coming out of the ground) also "scale" over at least a ten-fold range. Much more biological research is needed on anatomical scaling.
Another big concept, related to symmetry, is stability:
Divergence away from a stable state: Can break symmetry.
Is achieved either by positive feed-back, or loss of negative feedback
Convergence toward a stable state: Can create shape, & create symmetry
Is best achieved by negative feed-back, homeostasis
How to "break symmetry"
In order to form an animal body, starting with a sphere (or from a disk) (Both of which have infinite numbers of planes of reflection symmetry)(e.g. oocyte stage for most kinds of animals; and continuing as late as the blastula stage or its equivalent for embryos of many kinds of animals, including humans)
-
some method is needed to "Break" symmetry.
-
[Very important! Somewhat difficult.]
B) and then causing the right and left halves to become anatomically different from each other, aorta goes toward left, etc.)
Those are two important example of breaking symmetry.
Most of the research on the former (A) happens to have been done using frog and salamander one-cell-stage embryos; also brown alga, kelp such as Fucus, 1-cell-stage.
(Although there are hundreds of other examples, only a few of which have been studied)
In Fucus, the root-leaf axis (which they call by another name, but same meaning) of a spherical cell can be created by light direction, pH gradients, voltage gradients, and a dozen or so other directional cues. For example, the future root sprouts on the darker side of the spherical one-cell stage, unless this directionality is somehow over-ruled by an electric field, or one of the other directional triggers. Many facts about this creation of oocyte directionality were discovered by Prof. Ralph Quatrano, who was chairman of this department for many years in the 1980 and 90s. This work was done during that time by him and one graduate student in particular, whose name deserves to be included here (& will be as soon as I find or remember it.)
In embryos of frogs and salamanders the plane of right-left reflection symmetry is selected from the infinite number of alternatives by the location where the sperm enters the oocyte. This location becomes what seems to me the anterior, but to most people seems like the dorsal, side. (This is not a disagreement about facts, just words, and so I recommend you get used to calling it the dorso-ventral axis, in case it turns up on Med School entrance exams). The grey crescent is caused to form 180 degees opposite to where the sperm enters; and that causes the blastopore to develop at or near the grey crescent.
As I mentioned previously: The direction of gravity can also create this breakage of symmetry. Centrifugation can also over-rule or superimpose itself on the symmetry symmetry-breaking of frog and salamander 1-cell stage embryos.
Please consider the analogy to a old-style punching-dummy in the drawing below. I had one of these things when I was about 4 years old; it helped me understand physics. Those dummies were (are?) made with an approximately hemispherical bottom, and with a metal weight at the bottom center.
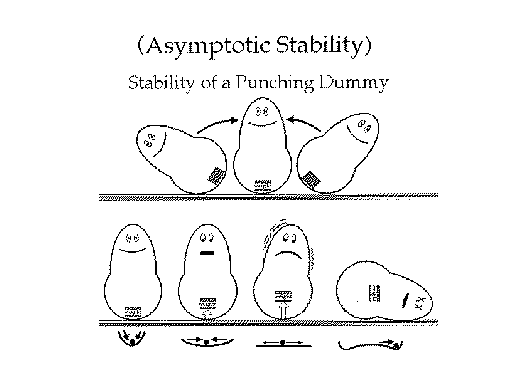
When you punch the top part, the whole upper part rolls away from you, and then rolls right back. The reason is not difficult to visualize. Because the weight is below the center of curvature of the hemispherical bottom, pushing the dummy to any non-vertical angle has the effect of raising the weight slightly. Gravity therefore pulls the axis back toward the vertical orientation. I mean this to be an illustration of how a system can gravitate toward having some particular orientation, or any other property. The term asymptotic stability is used by mathematicians. The counter-balanced forces can perfectly well be weights, springs, motors, or muscles. Lots of people mistakenly equate this kind of stability to minimization of thermodynamic free energy (Which is a magical incantation to them; that they honestly believe.)
Next, visualize how the dummy's behavior would change if some kind of little internal elevator lifted the weight As the weight approached the center of curvature, this would reduce how strongly the dummy would resist pushing, and how strongly it would gravitate back toward the vertical orientation.
If the weight were raised all the way to the center of curvature, this would eliminate the resistance to pushing, and also remove the tendency to roll back toward the vertical. It would behave like a basketball, with no more tendency for one side to be up, in preference to any other side being up. No stability
Finally, what will happen if your small imaginary elevator lifts the weight above the center of curvature. That would make the dummy unstable It would roll over in a random direction, and just lie there. If a fly had landed on one side, just at the moment when the weight rose past the center of curvature, then the dummy would have fallen toward the side with the extra weight (of the fly). Alternatively, if even a very weak wind had blown on the dummy, that tiny force would have become able to push the dummy over in the direction toward which the wind had blown.
The same result could be produced by the right kind of re-shaping of the curvature of the bottom, so that its center of curvature was shifted downward, below the weight. Please don't worry if you have trouble visualizing this; but give it a try. Standing up in a canoe is another good example of raising a center of weight above a center of (in that case) buoyancy.
What is the point of this punching dummy, and its change in behavior that changes so much depending on where the weight is relative to the center of curvature? It is meant to give you a mental framework for visualizing what sort of properties are needed to break symmetry
Something in the oocyte or the embryo needs to reduce its stability toward neutrality, and then slightly beyond
How should scientists try to understand symmetry breaking causes, like sperm entry, gravity, or the direction of flagellar waves? (which turns out to be the trigger for initiating right-left asymmetry in the human body, and probably all mammals and birds).
The usual perspective (of which the textbook is a good example) is to focus only on the movements in the cytoplasm, the redistribution of signal proteins (like Sonic Hedgehog), and particular genes whose mutation can cause failure of the system. (including "Lefty" and any of a long series of genes that code for component proteins of flagella.
Please do not interpret the next question as sarcasm: Please consider whether a molecular perspective is equivalent to trying to understand why the punching dummy fell over spontaneously by finding out what kind of plastic the dummy is made of, and whether the weight is made of lead or iron? (Likewise, in the case of not standing up in a canoe, is the key question whether the canoe is made of wood, aluminum or plastic? Or is the most important question the height of the person standing up?
My point is that it is at least as important to ask:
* Why the parts of the embryo were previously stable?
** What changed to destroy this stability?
*** And some other causal questions, too? Please suggest some.
Serious attempts to pose these questions in answerable forms were made by C. H. Waddington and Rene Thom and others during the 1960-70s and later. (Including several great Russian mathematicians: of these, Vladimir I. Arnold died recently. This approach was at the heart of what Thom called "Catastrophe Theory", (in the sense of abstract analysis of what makes systems undergo large-scale spontaneous changes). All big self-caused jumps in geometry or any property were called "catastrophes". Not enough research scientists had the patience to follow the logic. The approach wasn't disproved, or anything like that; It just didn't make enough useful or unexpected predictions to keep enough people interested.
I can tell you from experience, my only success getting most scientists follow a complex line of reasoning is to produce dramatic and unexpected photographs or time lapse videos that shake the out of their habitual assumptions.
It isn't enough to tell Horatio that there are more things in Heaven and Earth, you also need polaroid photos of the Ghost.
Otherwise people not only prefer to analyze what kind of wood canoes need to be made of in order to turn over, which makes them proud of being more "practical' and "molecular".
web sites about symmetry:
http://mathworld.wolfram.com/DualityPrinciple.htmlThis is an intelligent and accurate web site about many applications of symmetry (even to music!). You are not assigned to read it, but it could help you understand the topics covered in lecture and on the course web site.
The subject of symmetry is very wide and deep - like the Grand Canyon. So take a few minutes to look down into its beautiful depths, and even spend five or ten minutes hiking down in there to expose your mind to more profound concepts. Personally, I have been lucky enough to climb quite far. In additional to the conceptual beauty, you can take pleasure in the fact that you definitely won't need to know so much on exams in this course. We definitely are dealing with true profundity here, not pseudo-wisdom.
But if you have plenty of extra time and more brains than you know what to do with, try these:
http://en.wikipedia.org/wiki/Fractal
http://en.wikipedia.org/wiki/Circuit_theory-
http://en.wikipedia.org/wiki/Noether%27s_theorem
These are all completely accurate, as far as I can tell. Also well explained.
A major embryological question is "How do embryos manage to become less symmetrical " Break Symmetry" but avoid becoming random.
Sometimes symmetry breaking is accomplished by external stimuli:
A good example is that the anterior-posterior (most people say dorso-ventral)body axis of frog and salamander embryos isstimulated to form by the location where the sperm enters the egg cell at fertilization. You should learn dorso-ventral. This isn't a dispute about facts, but a preference in how to visualize things.
Whichever side the sperm enters is thereby caused to develop into the rear endof the future frog or salamander.
Until then, the oocyte had an infinite number of planes of mirror-image symmetry, all intersecting along a straight line that runs between the animal pole and the vegetal pole.
Sperm entry triggers a massive, activecortical rotation of thelayers of oocyte cytoplasm oocyte cortex relative to the deeper underlying cytoplasm, and in some species a "Grey Crescent" forms on the opposite side, below the equator. This area is visibly grey in color only in some species, but always has special properties. One very clever way to studycortical rotation is to apply spots of two different fluorescent dyes, one of which binds to molecules on the surface, and the other of which diffuses down into the deeper cytoplasm. The spots of surface-bound dyes slide dramatically past the spots of dye that have diffused down into deep cytoplasm. I really envy and admire the inventor of this experiment. We should draw a diagram of how it works. Or maybe draw an animation!
The location of sperm entry also controls the anterior-posterior axis of nematode worms. This was discovered by Prof. Goldstein of the UNC Biology Department!
What would you predict would happen if frog oocytes were fertilized exactly at their animal poles? In other words, what if no directionality were provided by the location of sperm entry?
One possibility: A very abnormal tadpole would develop which is either all mouth or all tail, 360 degrees around its periphery, or in some other way retains all of its million planes of reflection symmetry?
Another possibility: Another, unknown, or random disturbance would trigger a cortical rotation that is indistinguishable from cortical rotations that are stimulated by sperm entry.
This reminds me of a joke about some change being so hyper-sensitive that it will occur "not only at the drop of a hat, but in the presence of a loosely-held hat"
This degree of hypersensitivity runs the risk that two different body axes will be stimulated to form, in the same oocyte. (conjoined twins). This can be caused to occur simply by holding a recently-fertilized frog oocyte upside down (animal pole down). The higher density and weight of the vegetal pole cytoplasm shifts downward and this causes formation of two grey crescents, and thus two separate blastopores on opposite sides of the embryo. This was originally discovered in the 1890s, and then re-discovered in the 1980s.
new material added to this page January 21:
How Symmetry Relates To Embryology
1) Embryology tries to understand how genes create and maintain anatomical shapes and patterns.2) (Usually) Objects have the same symmetries (or sometimes more symmetry) as their causes. (This generalization is called Curie's Principle.)
3) For example, spherical shapes result from counter-balances of forces which have the same strengths in all directions.
4) Notice that similarity of shape between two objects does not require or imply that they are caused by the same forces; It implies that the symmetries of the forces are the same, or similar.
[Incidentally, in my opinion D'Arcy Thompson's big mistake was to think that similarity of shapes implies that the same actual forces cause both shapes: For example that similarity to the shapes of soap bubbles implies that cells are shaped by "surface tension", instead of by some force that contracts equally strongly in all directions. Active contractility of acto-myosin layers near cell surfaces can pull with the same strength in all directions; So can elastic stretching, and who knows how many other forces.
Similarity of shape implies similarity of causal forces, and does NOT imply that the actual forces are the same. This mistake holds back progress. Many examples of this mistake have occurred in embryology, and I will point them out as we go along.]
5) To "break" symmetry means to cause an object or structure to become less symmetrical.
What is broken is Curie's Principle. Several important phenomena are examples of breaking symmetry. One example is using the location of sperm entry to decide where the plane of right-left reflection symmetry will be located. Another example of symmetry breaking is using flagellar basal bodies to decide differences between right and left. A third example is use of "Reaction-Diffusion Systems" to break displacement symmetry, in the sense of creating stripes, spots, somites, or other spatially periodic patterns.
6) Hermann Weyl defined different kinds of symmetry in terms of what reflections, rotations, displacements, enlargements or distortions will cause an object or pattern to look the same as it did before. For example A, B, C, D and E all have one plane of reflection symmetry; H and I have two planes of reflection symmetry; A square has four planes of reflection symmetry, a triangle has three, a rectangle two, and starfish 5. N and two other letters each have one axis of two-fold rotation symmetry. Flagellar basal bodies have 9-fold rotational symmetry, if we ignore the central pair of microtubules.
Normally-proportioned half-sized embryos, quarter-sized, or double sized embryos of sea urchins can develop from separating the first two cells, or the first four cells, or from fusing two embryos. We could have called this two-fold, eight-fold etc. magnification symmetry (or "enlargement" or "shrinkage" symmetry), but the physicists got there first and call this sort of thing "dilation symmetry" (symmetry with respect to change in size).
7) One of the 2 or 3 deepest questions in developmental biology can be phrased as "How do early embryos accomplish dilation symmetry". It must be because their fundamental control mechanisms have dilation symmetry.
Lewis Wolpert's concept of "Positional Information" boils down to two plausible mistakes: One is that only diffusion gradients have enough dilation symmetry to accomplish size "regulation" (as in the example of separating early embryonic cells); The other mistake is that all cause and effect phenomena amount to information transfer.
We will learn that Dictyostelium fruiting bodies accomplish at least a hundred-fold dilation symmetry. Rounding up of liquid drops and soap bubbles also has dilation symmetry, and so does formation of meanders in rivers. There are many examples of dilation symmetry, besides diffusion gradients.
8) Fractals are another interesting category of dilation symmetry. Each part of a fractal pattern is a miniature scale model of the entire pattern. The branching pattern of the lungs are an example of a fractal. Embryologically, lungs form as two small out-pocketings of the wall of the endodermal tube. The epithelial walls of these out-pocketings fold actively (driven by contraction of cytoplasmic acto-myosin) so as to subdivide themselves into two pockets. Each of these pockets then repeats this process of subdivision into two, and this doubling process is repeated again and again, creating first 2, then 4, then 8 then 16, 32, 64 etc. out-pocketings. In humans, this process of repeated doubling continues until a million small epithelial pockets have been formed, each one of which then becomes a thin-walled alveolus. The lungs of all mammals have alveoli, but lung structures are significantly different in birds, reptiles and amphibians. For example, birds have "air capillaries", which are epithelial tubes that extend around in loops, so that air can flow continually in one direction, instead of in and out.
Other organs also form by sequential branching of epithelial tubes. Salivary glands, the pancreas, the liver, and ducts inside kidneys form this way. During this branching process, you could hardly distinguish between developing lungs, developing salivary glands, pancreas or kidneys. A time-lapse video of kidney development will be shown in class, but cannot be incorporated into the course web pages, because I didn't make it, and a publisher owns the copyright.
The Mandelbrot set is one of many mathematical fractal patterns that are comparatively easy to generate by repeated recalculations. The bright-colored blobby pattern at the beginning of our web pages about symmetry is a tiny part of the Mandelbrot set, which was generated on my Mac lap-top by repeated squaring of complex numbers (those numbers that include the square root of minus one). Repeated cubing will also generate fractal patterns, and so will repetition of other processes, whether mathematical or physical (as in the lungs). Tidal rivers and creeks develop fractal branching patterns. You can see pictures of them using Google Maps. Good examples are the rivers east of Elizabeth City and in Currituck County, North Carolina. The North River is a good example.
9) A good way to discover the cause of any self-forming pattern is to consider its symmetry, and remember Curie's Principle. If a pattern has a certain kind of symmetry, then expect that the causes of this pattern either have that same kind of symmetry, or that "Symmetry Breaking" occurs, in the sense of external triggers being used to reduce symmetry.
Examples of symmetry breaking include processes that from any other point of view wouldn't seem to have anything fundamental in common. Locations of sperm entry and directions of gravity are used to "break" the axial symmetry of newly fertilized one-cell stage embryos. Reaction-diffusion systems break displacement symmetry, in the sense of producing waves of alternating high and low chemical concentrations.
Elongation of cartilages is driven by osmotic swelling of cartilage. Osmotic pressure is a scalar variable, and therefore incapable of being stronger in one direction than another. So how can it make femurs (etc.) elongate directionally? Because collagen fibers wrapped around and within growing cartilages channel the osmotic pressure into certain directions, by preventing expansion in other directions. Tension, being a tensor, can and does vary with direction.
Thus the reduced symmetry of skeletal shapes (that is, its directionality) is produced by directional differences in tensions that resist swelling. The swelling force itself is incapable of directionality. Textbooks in anatomy and histology rarely have a clue on this subject, and assert that cartilage elongation and shape is produced by chondrocytes pushing harder in certain directions than others. In histological sections, you can see the chondrocytes lining up in the direction of greatest pressure. But its not them that's pushing harder in that direction. Neither the cells nor the cartilage matrix are capable of exerting more force in one direction than another. Both the cells and the extracellular matrix line up in the direction of least resistance (specifically, resistance by tension in collagen fibers).
Good luck trying to get biomechanics specialists to understand this, however. It's too simple for them.

10) Regarding whether reaction-diffusion systems are really how embryos generate spatial patterns (like zebra stripes and leopard spots) one of the best counter-arguments is based on the lack of dilation symmetry. Specifically, how do the sizes of the waves adjust in proportion to increased or decreased sizes of the tissue to be subdivided.
A (barely) conceivable method would be for the ratio of diffusion constants to change in proportion to the size of the material available. This would cause the wavelength to change. The larger the ratio of diffusion constants, the narrower and closer together the stripes; the smaller the ratio, the wider and farther apart the waves. Changing the wave lengths could also be accomplished by the right changes in the chemical reaction rates.
These methods feel contrived, however. It's hard for me to believe in them.
Color patterns in certain species of fish don't change their wave-length in proportion to size. As these fish grow larger, these fish keep adding new stripes. This observation has been widely interpreted to be evidence specifically in favor of reaction-diffusion systems. This tacitly assumes that reaction-diffusion systems are the only possible way to break displacement.
Many animals have alternating wide and narrow stripes. Not a few have more complex patterns of alternation, of which my favorite is wide / very narrow/ intermediate width / very narrow / very wide, and repeat. Look at the stripes on these dreadful Lion Fish that have unfortunately transplanted to the Atlantic and Caribbean, of which there are several photographs in the west hallway in Coker Hall.